Exponential and logarithmic functions provide a structured way to describe growth, calculate interest rates, understand elasticity, and analyze many other aspects of economic behavior. While these functions might seem intimidating at first, their applications in economics are both practical and insightful. In this blog post, we will explore how exponential and logarithmic functions are used in economic analysis, ranging from modeling growth rates to calculating the effects of compound interest.
What are Exponential and Logarithmic Functions?
Before diving into their applications, let’s clarify what exponential and logarithmic functions are:
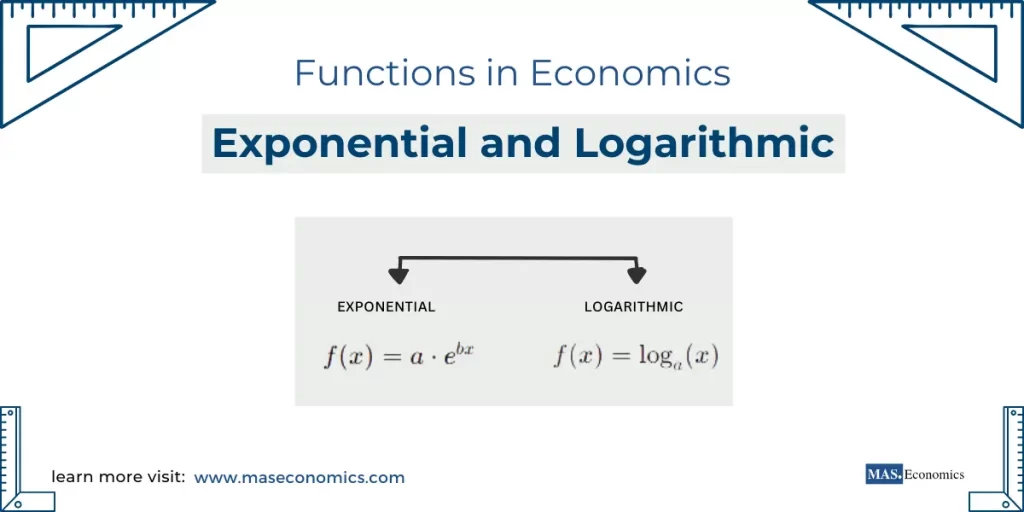
Exponential Function: An exponential function takes the form:
Logarithmic Function: The logarithmic function is the inverse of the exponential function. A common form is:
Exponential Growth in Economics
Modeling Population and Economic Growth
One of the most common uses of exponential functions in economics is in modeling growth, whether it’s the population of a country, the value of an investment, or the GDP of an economy. When we say that a quantity grows exponentially, we mean that it increases by a fixed percentage over equal time intervals. This type of growth is characterized by the equation:
In which:
- \(P(t)\): The population or value at time \(t\).
- \(P_0\): The initial population or value.
- \(r\): The rate of growth.
- \(t\): Time.
For example, if the GDP of a country is growing at a rate of 3% per year, we can use an exponential function to project future GDP. Exponential growth models are particularly important when assessing the long-term impact of policies or investments. They show us how small changes in growth rates can lead to significant differences over time, illustrating the power of compounding.
Compound Interest and Investment Returns
Another key area where exponential functions come into play is in the calculation of compound interest. If you invest an initial amount of money \(P_0\) at an interest rate \(r\) that compounds continuously, the value of the investment at time \(t\) is given by:
This formula illustrates how investments grow over time when the interest earned itself earns interest—a concept known as compounding. Understanding this principle is fundamental for financial planning, as it highlights why starting to invest early can be incredibly advantageous. For example, even a small difference in the interest rate or an additional year of investment can make a substantial impact on the final return due to the exponential nature of compounding.
Logarithms in Economic Analysis
The Concept of Elasticity
Logarithmic functions are frequently used in economics to understand elasticity, which measures the responsiveness of one variable to changes in another. The most common type is price elasticity of demand, which shows how the quantity demanded of a good responds to changes in its price. The formula for elasticity often involves logarithms to help convert percentage changes into more manageable calculations:
Using logarithms simplifies the process of calculating elasticity because it turns multiplicative relationships into additive ones, which are much easier to differentiate and interpret. Essentially, logarithmic transformations help economists linearize data, making it simpler to identify the relationships between variables.
Diminishing Returns and Utility Functions
Logarithms also appear in utility functions, especially those that exhibit diminishing marginal returns. A common utility function that incorporates logarithms is:
In this case, \(U(x)\) represents the utility (satisfaction) that a consumer derives from consuming \(x\) units of a good. The logarithmic form suggests that each additional unit consumed adds less to total utility than the previous one—a concept known as diminishing marginal utility. This kind of modeling helps explain why consumers diversify their purchases rather than spending all their income on a single good.
The Connection Between Exponential and Logarithmic Functions
One of the most powerful aspects of using exponential and logarithmic functions in economics is their inverse relationship. This relationship allows economists to solve equations involving growth and decay efficiently. For instance, if you know the future value of an investment and want to calculate the rate of growth, you can use logarithms to solve for the rate \(r\).
Consider the compound interest formula again:
To solve for the rate of interest \(r\), we take the natural logarithm of both sides:
Rearranging gives:
This ability to switch between exponential and logarithmic forms makes these functions incredibly versatile, whether you are projecting growth or assessing the impact of different factors on economic outcomes.
Real-World Examples
Predicting Long-Term Economic Growth
Governments and international organizations often use exponential functions to predict long-term economic growth. For instance, if a developing nation is experiencing a 5% growth rate, the power of exponential modeling allows policymakers to estimate when the nation’s GDP will double. By applying the Rule of 70 (which is derived from the natural logarithm), we can approximate the time it will take for the GDP to double:
In which \(r\) is the annual growth rate percentage. In our example, it would take approximately \(\frac{70}{5} = 14\) years for the GDP to double.
Assessing the Impact of Inflation
Exponential functions are also used to model inflation, showing how the purchasing power of money decreases over time. For example, if the inflation rate is constant at 2%, we can use an exponential function to predict how much a fixed amount of money will be worth in the future. This helps individuals, businesses, and governments make better decisions about spending, saving, and investing.
Conclusion
Exponential and logarithmic functions are indispensable in economics. They allow us to model growth, understand consumer behavior, and make predictions about future economic outcomes. By converting complex relationships into manageable forms, these functions make it possible to see the bigger picture and make informed decisions based on mathematical analysis.
FAQs:
What are exponential and logarithmic functions, and how are they used in economics?
Exponential functions model growth and decay, often used for projecting GDP or population growth. Logarithmic functions, being the inverse of exponential functions, help convert multiplicative relationships into additive ones, simplifying calculations in elasticity and utility analysis.
How do exponential functions model economic growth?
Exponential functions represent how values grow by a fixed percentage over time. For example, GDP growth can be modeled using the equation \(P(t) = P_0 e^{rt}\), where \(P(t)\) is the GDP at time \(t\), \(P_0\) is the initial value, \(r\) is the growth rate, and \(e\) is Euler’s number. This function highlights the power of compounding over time.
How is compound interest calculated using exponential functions?
Compound interest follows the formula \(A(t) = P_0 e^{rt}\), where \(A(t)\) is the value of the investment at time \(t\), \(P_0\) is the initial amount, \(r\) is the interest rate, and \(t\) is time. This formula shows how continuous compounding accelerates investment growth over time.
What role do logarithmic functions play in elasticity calculations?
Logarithmic functions help linearize complex relationships, making it easier to measure elasticity. For example, price elasticity of demand is calculated using the formula \(E = \frac{\partial \ln Q}{\partial \ln P}\), where \(Q\) is quantity demanded and \(P\) is price. Logarithmic transformations simplify working with percentage changes.
How do logarithms help explain diminishing marginal utility?
Logarithmic utility functions, such as \(U(x) = \ln(x)\), capture diminishing marginal utility, indicating that each additional unit of consumption increases utility by a smaller amount. This model explains why consumers spread their spending across multiple goods rather than concentrating on one.
What is the inverse relationship between exponential and logarithmic functions, and why is it useful?
Since logarithms are the inverse of exponentials, they allow economists to solve for unknown growth rates or time. For example, if \(A(t) = P_0 e^{rt}\), taking the natural logarithm of both sides gives \(\ln(A) = \ln(P_0) + rt\), which can be rearranged to solve for \(r\).
How do economists use exponential functions to predict long-term growth?
Economists apply exponential models to forecast GDP growth or population increase using the Rule of 70, which estimates doubling time with \(T \approx \frac{70}{r}\), where \(T\) is the doubling time and \(r\) is the annual growth rate percentage. This tool helps policymakers plan for future needs.
How are exponential functions applied in inflation modeling?
Exponential models help with inflation predictions by showing price growth over time. For instance, with a constant rate of 2%, the formula \(P(t) = P_0 e^{0.02t}\) shows how the value of money decreases over time as prices increase with continuous compounding.
Why are exponential and logarithmic functions critical in economic analysis?
Exponential and logarithmic functions are applied to model growth, understand elasticity, and forecast trends with mathematical precision. They simplify complex relationships, allowing for clearer insights into economic behaviors and policies.
Thanks for reading! Share this with friends and spread the knowledge if you found it helpful.
Happy learning with MASEconomics